Curs engleză

The Math Behind the AHP

6.1. Decision matrices

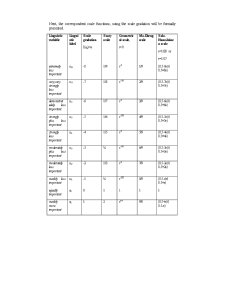
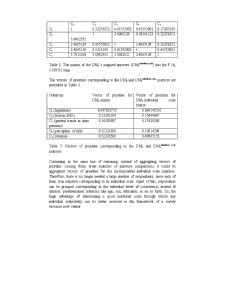
A decision matrix represents a square matrix whose elements are interpreted as being pairwise comparisons among criterions or alternatives. Initially these comparisons are done in verbal terms, and then these verbal terms are put in correspondence with numerical values through a so called scale function. The number of verbal comparisons is set at the beginning by the researcher who controls the experiment. Secondly, the researcher has also to justify the choice of a certain scale function against other options.

Suppose that in general n-criterions C1,..Cn have to be compared by a certain decision maker, two by two. The result of this comparison is a numerical (n,n) matrix with positive elements, referred as a decision matrix and denoted with DM:

If criterion Ci is preferred to criterion Cj with certain intensity measured on a numerical scale, this will be registered in the decision matrix as being the element aij and it will be placed on the line i and column j.

If criterion Ci is preferred to the criterion Cj it means that the numerical value associated to the intensity of this preference, referred as aij has to be greater than the numerical value associated to the intensity of preference of Cj over Ci, referred as aji. The exact relation among these intensities of preferences is assumed to be reciprocal, or in other words the reciprocity condition is assumed to hold true

(6.1-1)

Also, it is assumed that one is indifferent in choosing among Ci and Ci and therefore the associated intensity will be one. This reflexivity condition translates into

(6.1-2)

Therefore, a decision matrix is a square matrix with positive elements satisfying conditions (6.1-1) and (6.1-2).

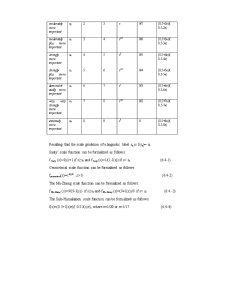
If criterion Ci is preferred to criterion Cj with intensity aij and if criterion Cj is preferred to criterion Ck with intensity ajk then consistency assumes transitivity of preferences and proportionality of the corresponding intensities. So, the consistency condition is

(6.1-3)

One can keep in mind that while the term decision matrix translates into a square, positive matrix checking reciprocity and reflexivity conditions (4.4.1-1) and (4.4.1-2), the consistency condition may or may not be fulfilled.

Some remarks can prove useful in becoming confortable with the previous definition.

Remark 1. A positive (2,2) matrix is a decision matrix which is automatically a consistent one.

Remark 2. A positive (3,3) matrix is a decision matrix which is consistent if and only if

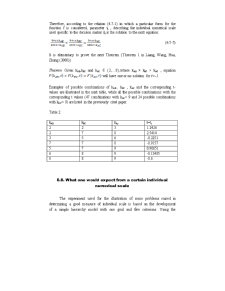
6.2. Priority vectors

A priority vector , associated with a (n,n) decision matrix DM is the eigenvector associated with the highest (real number) eigenvalue, noted with .

In general, if A is a (n,n) matrix, the eigenvalues ( ) are (the real numbers ) solutions to the so-called characteristic equation

(6.2.-1)

The characteristic equation (6.2-1) is a n-degree equation in and therefore it has at most n real solutions. The highest solution is referred with .

If is an eigenvalue associated the matrix A, then the eigenvector

associated with is the solution to the matrix equation

(6.2.-2)

Using the previous notations, the following result holds true:

DM is a (n,n) consistent decision matrix if and only if =n.

If DM is a (n,n) decision matrix then .